Solutions to Logic Problems by Michael Keller

Euchre

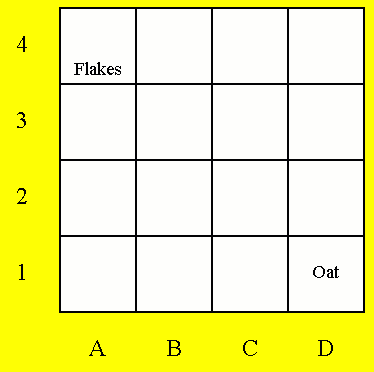
Joe held a black jack (clue 2) but not the jack of clubs (clue 3), so he held the jack of spades and nine of clubs (clue 2). He also held the ten of hearts and ten of diamonds (clue 5). We have identified four of his six cards, so he cannot be Blackwood, who held four kings (clue 4), so Joe is Cavendish and David is Blackwood, (clue 4). Joe also held the queen of spades and queen of clubs (clue 4), so we have identified all six of his cards. Bob is not Foster (clue 6), so Bob is Phillips and Harry is Foster. David held the four kings (clue 4) and the jack of clubs (clue 3). He also held the queen of diamonds, since it was not held by Bob or Harry (clue 6), or Joe, whose six cards we already know. Bob held the queen of hearts (clue 4), jack of hearts (clue 3), and the three aces (spades, diamonds, clubs) not held by Harry (clue 4), so he also held the ten of clubs (clue 1). Harry held the ace of hearts (clue 4) and the remaining unnamed cards (jack of diamonds, ten of spades, nine of diamonds, nine of hearts, nine of spades).

Grandmaster Tournament:
For any two of the four characteristics held by three players, exactly one player must have both characteristics. Let's take Igor and Kuznetsov.   If two players were named Igor Kuznetsov, it would violate clue 4; if the three players named Igor and the three named Kuznetsov were six different players, it would be impossible to have three players from Moscow without having either two named Igor or two named Kuznetsov, and again clue 4 would be false.   So there is one player named Igor Kuznetsov.   Igor Kuznetsov cannot be from Moscow (since only one other player is named neither Igor nor Kuznetsov, and we cannot have three players from Moscow without contradicting clue 4.   For the same reason, we know Igor Kuznetsov does not prefer the Ruy Lopez.   In order to follow clue 4, the three players from Moscow must be one of the players named Igor and one of the players named Kuznetsov (but not Igor Kuznetsov in either case), and Dmitri Sergeev, who is the only player named neither Igor nor Kuznetsov. The same is true of the three Ruy Lopez players, and since Dmitri is from Moscow and likes the Ruy Lopez best, one of the players named Igor is from Moscow and the other prefers the Ruy Lopez, and the same is true of the players named Kuznetsov.  We can start to sketch out some of the facts we know:

Do we know who plays who yet?   Igor Kuznetsov cannot play any of the other players named Igor or Kuznetsov (clue 5), so he plays Dmitri.  The Kuznetsov from Moscow plays the player named Igor who prefers the Ruy Lopez, and vice versa.    From clue 2, we know that Igor Kuznetsov prefers the French, and is not from Omsk.  He isn't from Volgograd either (clue 3), so he is from St. Petersburg.   Let's pair up the players and add what we know:

Pavel is the Kuznetsov from Moscow, since he prefers the Nimzo-Indian (clue 6).   By elimination, Igor from Moscow prefers the Sicilian (and is Alexandrov, since he is not Petrov from clue 3).  His opponent Kuznetsov is from Volgograd (clue 3) -- and is Boris, by elimination.  The player from Omsk is Petrov, by elimination. The full solution:

Igor Kuznetsov played Dmitri Sergeev, Boris Kuznetsov played Igor Alexandrov, and Igor Petrov played Pavel Kuznetsov.

Cereal Display

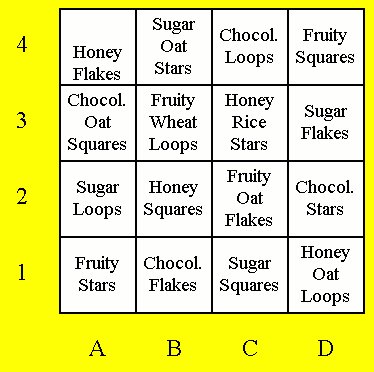
The grid above is labeled to make the solution easier to follow.

Sugar Oat Stars are on the top shelf (clue 1). Oat flakes are not on the bottom shelf (clue 4), so they are on the third shelf down (clue 8). Fruity Wheat Loops are on the second shelf down (clue 6), so the oat cereal on that shelf is oat squares, and the cereal at D1 is oat loops. Sugar Oat Stars are not at A4 (clue 7) nor B4 (clue 3), so they are at B4 or C4. Oat flakes are not at A2 or D2 (clue 3), so they are at B2 or C2, not aligned with Sugar Oat Stars. Oat Squares are therefore at A3 (clue 3), and they are Chocolate Oat Squares (clue 8). The oat loops at D1 are not fruit-flavored (clue 6), so they are honey (clue 5). The oat flakes are Fruity Oat Flakes (clue 5). The cereal directly above the Fruity Oat Flakes (at either B3 or C3) is not squares or loops, so it is stars. The cereal at D3 is therefore flakes, and neither chocolate, fruit-flavored, nor honey, so it is sugar. The cereal at C3 is rice (clue 2), so Fruity Wheat Loops are at B3 and Sugar Oat Stars at B4. Honey Rice Stars are at C3, and Fruity Oat Flakes are at C2. The rest of the shapes and flavors can be filled in using clues 3 and 5: for example, loop cereals are at A2 and C4, stars at A1 and D2, squares at B2, C1, and D4. Honey cereals are at A4 and B2, etc.

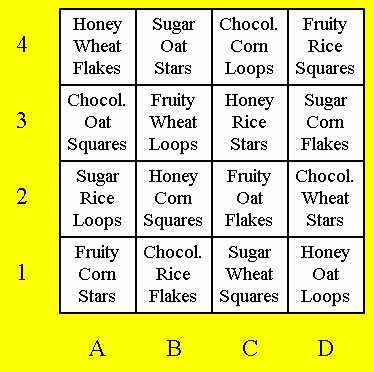
Now the wheat cereal in column A is not at A1 or A2 (clue 5), so it is at A4. The other wheat cereals are at C1 and D2. The cereal at C4 is corn (clue 3), and D4 is rice. The rest follows easily. The full solution:

This puzzle is an extended example of a Greco-Latin Square, an multiple arrangement of items so no element is repeated in the same row or column, and no two cells share more than one element in common.   Another kind of puzzle based on Latin Squares is Mark Thompson's Latin Squares.   Everett Kaser's site also has a good page on Mark's puzzle.   The most famous puzzle based on Latin Squares is Sudoku, a somewhat simpler relative of Mark's game.

Portions of this article previously appeared on the Games Cafe (www.gamescafe.com) in 2000; that site is no longer in operation.
This article is copyright ©2000, 2020 by Michael Keller.  All rights reserved.